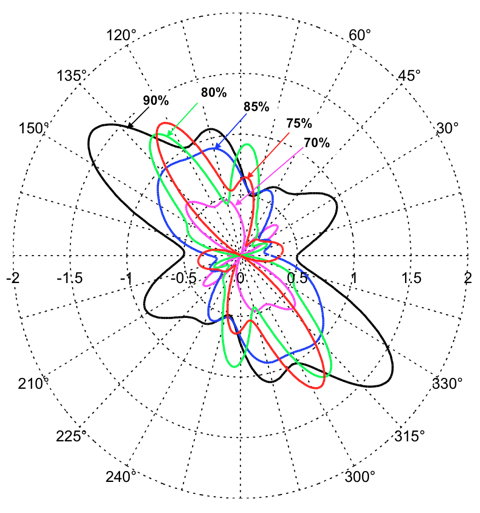
Evolution of micro-crack distribution of fiber micro-cracks using tenth order fabric tensor approximation of the [±45]s laminate

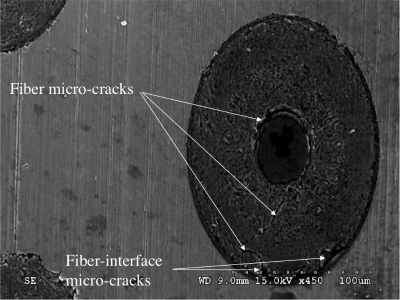
Fiber and fiber-interface micro-cracks in a 85% load [±45]s sample.

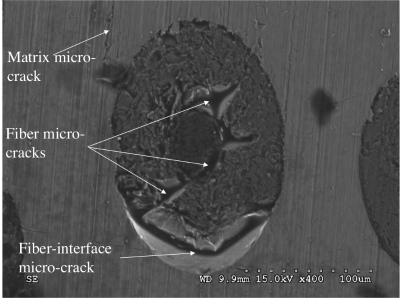
Fiber and fiber-interface micro-cracks in a 90% load [±45]s sample.

A New Fabric-Based Damage Tensor

In this fundamental and pioneering work in Solid Mechanics the concept of fabric tensors is used to characterize damage in composite materials. This is achieved within the framework of the theory of elasticity. A directional data model of damage mechanics for composite materials is developed using fabric tensors. The introduction of fabric tensors into the analysis of damage of composite materials allows one for an enhanced and better understood physical meaning of damage. The micromechanical approach is used here to relate the damage effect through fabric tensors to the behavior of composite materials. In this approach, damage mechanics is introduced separately to the constituents of the composite material through different constituents’ damage effect tensors. The damaged properties of the composite system as a whole can then be obtained by proper homogenization of the damaged properties of the constituents. The derivation of a generalized formulation of damage evolution is shown here in a mathematically consistent manner that is based on sound thermodynamic principles. Numerical examples are obtained to show applicability.

The exact mathematical relations between the damage tensor and fabric tensors are derived. These relations provide the missing link between the subjects of Damage Mechanics and Fabric Tensors. In this regard, a new fabric-based damage tensor is formulated. These tensors play an important role in the mechanical characterization of the micro-structure of materials. Thus solids with micro-cracks may be studied using the relations provided within the framework of Damage Mechanics. Contributions in this area include:

a fourth-rank damage tensor is used and its exact relationship to the fabric tensors is illustrated,
the definition of the fabric tensor incorporates both orientation and length of a micro-crack,
a model of damage mechanics for directional data is formulated using fabric tensors,
values of the fabric tensors and damage tensor are calculated for various micro-crack distributions with different orientations,
relations are derived explicitly for the general case of anisotropic elasticity with anisotropic damage,
four cases are solved using these relations, namely the cases of isotropic elasticity with isotropic damage, isotropic elasticity with anisotropic damage, plane stress, and plane strain,
micro-crack distributions of samples subjected to uniaxial loads of a metal matrix composite material reinforced with continuous fibers are measured experimentally, and then quantified using the fabric tensor approach,
samples loaded at different levels are tested to show how micro-crack distributions develop with applied loads,
the micro-cracks can be divided into two categories: fiber micro-cracks and fiber-interface micro-cracks,
fabric tensors of zero, second, fourth, sixth, eighth and tenth order are used to approximate these micro-crack distributions and they give a very good approximation when eighth or tenth order fabric tensors are used for both fiber and fiber-interface micro-crack distribution types.

A book is published in this area at the special invitation of a publishing company:

Voyiadjis, G. Z., and Kattan, P., Advances in Damage Mechanics: Metals and Metal Matrix Composites With an Introduction to Fabric Tensors (2nd edition), 742 p., Elsevier, Oxford, ISBN: 0-08-044688-4, 2006.